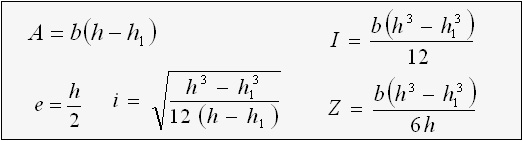Cross Section

A：Area （Units²）
e：Extreme point（Units）

I：Moment of Inertia（Units⁴）
Z：Section Modulus（Units³） →　I/e
i：Radius of Gyration（Units）　→ √（I/A）

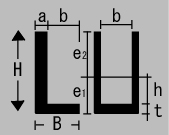
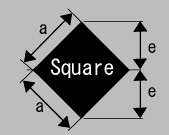
Square

e = a/2

Z = a³ /6

i = a / √12 = 0.28867a

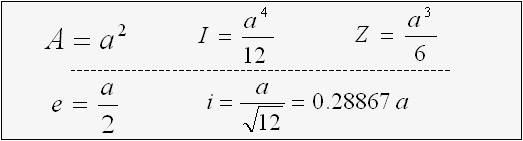
Square

e = a / √2

Z = a³ / ( 6√2 )

i = a / √12 = 0.28867a

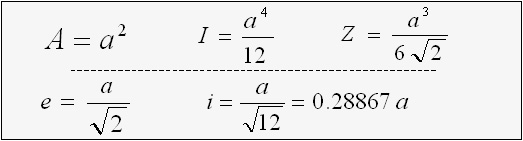
Rectangle

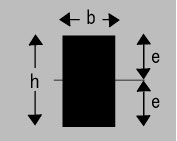
e = h / 2

Z = bh² /6

i = h / √12 = 0.28867h

Rectangle
at Angles

e = bh / √( b² + h² )

Z = b² h² / ( 6 √( b² + h² ) )  

i = b h / √( 6 ( b² + h² ) )   

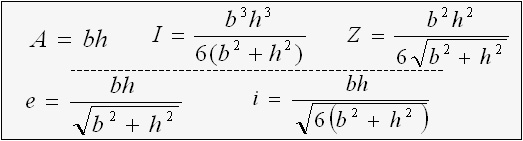
Rectangle
at Specified Angles

e = ( h・cosθ + b・sinθ) / 2

Z = b h ( h²・cos²θ + b²・sin²θ) / ( 6 ( h・cosθ + b・sinθ ) )

i = √( ( h²・cos²θ + b²・sin²θ) / 12 )   

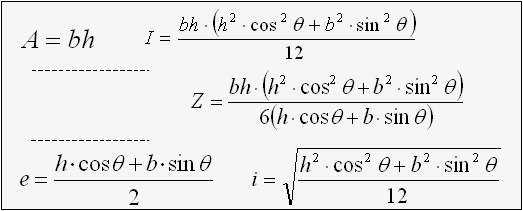
Square Tube

e = a / 2

Z =( a⁴ - a1⁴ ) / ( 6a )

i = √( ( a² + a1² ) /12 )

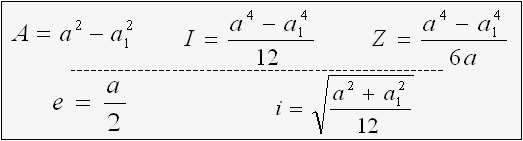
Rectangle Tube

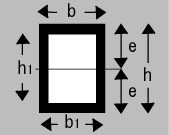
e = h / 2

Z = ( bh³ - b1h1³ ) / ( 6h )

i = √(( bh³ - b1h1³ )/ ( 12(bh - b1h1 )))

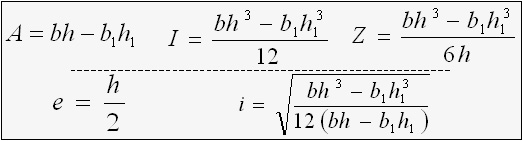
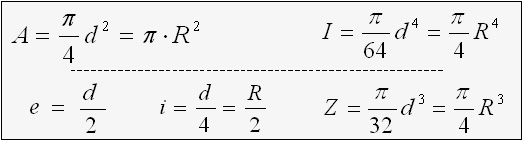
Round

e = d / 2

Z = πd³ / 32 = πR³ / 4

i = d / 4 = R / 2

Round Tube / Pipe

e = D / 2

Z = π( D⁴ - d⁴ ) / 32D

i = √ ( D² + d² ) / 4

H ・ C

Section of the same group
Part-１

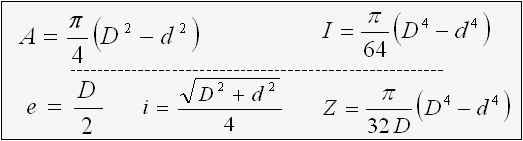
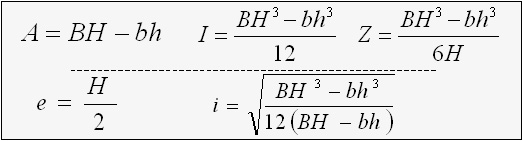
e = H / 2

Z = ( BH³ - bh³ ) / ( 6H )

i = √( ( BH³ - bh³ )/ ( 12( BH - bh )))

H ・ T

Section of the same group
Part-２

e = H / 2

Z = ( BH³ + bh³ ) / ( 6H )

i = √( ( BH³ + bh³ )/ ( 12( BH + bh )))

L ・ U

Section of the same group
Part-３

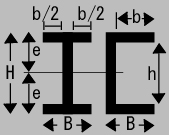
e1 = (aH² + bt²) / ( 2(aH + bt))

e2 = H - e1

Z = I / e1 　：　Z = I / e2

i = √( I / A )

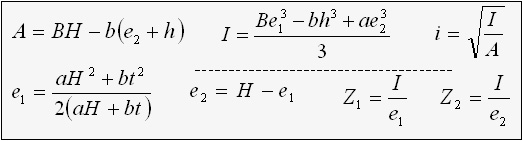
H

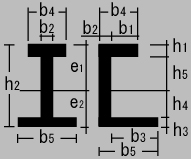
e1 = h2 - e2

e2 = (b2h2² + b3h3² + b1h1( 2h2 - h1)) / ( 2 (b1h1 + b2h2 + b3h3 ))

Z = I / e1 　　：　Z = I / e2

i = √( I / A )

Top and Bottom
is not same

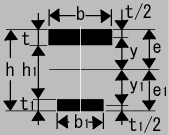
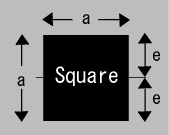
e = (0.5bt² +  b1t1 (h-0.5t1)) / A

e1 = h-e

Z = I / e 　　：　Z = I / e1

i = √( I / A )

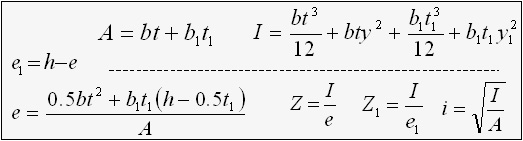
Top and Bottom
is same

e = h / 2

Z = b ( h³ - h1³ ) / ( 6h )

i = √(( h³ - h1³ )/ ( 12(h - h1 )))